
April has been Mathematics Awareness Month since 1999, making this the nineteenth time it's been observed. Wait, that's not right. The twentieth time. To celebrate our mathematical awareness, or lack thereof, Ken Jennings of Jeopardy! will be with us all month debunking all the popular misinformation about numbers that you thought once you could count on.
The Debunker: Did the Greeks Love the "Golden Ratio"?
The "golden ratio" is an irrational number approximately equal to 1.62, and it's a relationship easy to find in many natural sequences and geometries. Take two line segments, a longer one and a shorter one. The two lengths are in the "golden ratio" if the ratio of the shorter to the longer is the same as the ratio of the longer to the sum of both segments together. Readers of pop mathematics are probably familiar with claims that artists and architects have always loved the golden ratio, and that it can be found by measuring everything from the Pyramids to the Parthenon in Athens to the face of the Mona Lisa. In Dan Brown's The Da Vinci Code, the golden mean (which he calls "phi," a Greek letter sometimes used to represent the ratio) provides pivotal clues to the mystery, and is said to underlie a lot of great classical and Renaissance art.
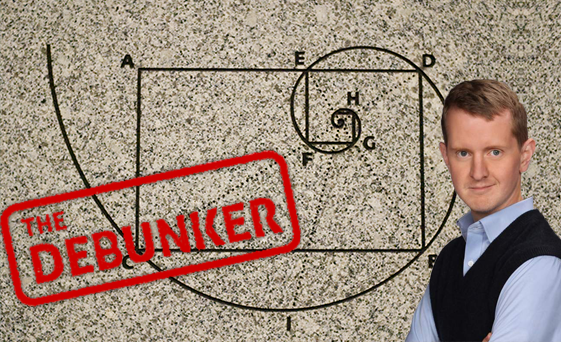
It might come as a surprise, then, to know that this is essentially all conjecture. There is no evidence whatsoever that the Greeks considered the golden ratio aesthetically pleasing, or used it in their art and architecture at all. Euclid does mention the ratio as a geometric construction in his book Elements, but he never calls it "golden." The idea that the ratio is some perfect "divine proportion" may date back no farther than the Renaissance, when mathematician Luca Pacioli wrote about it in De divina proportione. And the Greek letter phi wasn't associated with the ratio until Mark Barr, an American mathematician, proposed it in the early 20th century.
So the next time you see a photograph of the Parthenon that someone has superimposed the "golden rectangle" upon, please note that this is an idea that only dates back to the 1850s. In fact, the Parthenon takes its harmonious appearance from the clever deployment of lines that look parallel but in fact converge or curve, so it's virtually impossible to take measurements precise enough to nail down ratios. Any resemblance to the ratio Euclid wrote about a century later might be pure conjecture.
Similarly, Leonardo da Vinci's Vitruvian Man diagram is often taken as a demonstration of "phi" at work—and indeed, the naked fella's navel is at a plausible "golden ratio" relative to his height. But da Vinci's notes clearly lay out the proportions he's illustrating, and the math behind the drawing is all based on whole numbers. He never mentions the golden ratio at all. Why would Leonardo rely on the ineffable beauty of the golden mean to drawn the human figure…but then not tell us about it? If you're Dan Brown, the answer might be, "Because he was laying a diabolical series of clues to an occult mystery!" But if you're using Occam's Razor, the answer is probably, "Because he wasn't thinking about the golden ratio at all."
Quick Quiz: The name "phi" might have been chosen because it suggests what Italian mathematician, whose namesake sequence expresses the golden ratio?
Ken Jennings is the author of eleven books, most recently his Junior Genius Guides, Because I Said So!, and Maphead. He's also the proud owner of an underwhelming Bag o' Crap. Follow him at ken-jennings.com or on Twitter as @KenJennings.
